Title: The geometry of level sets
Julie Clutterbuck, Monash University
Abstract: The level set of a function $u$ is the curve or surface $u^{-1}(c)$. If a positive function is a solution to an elliptic equation on a bounded domain, the shape of its level sets are necessarily constrained. Investigating the shapes of the level sets has been a fruitful strand in PDE over the last few decades, leading to tools that are used in many applications. The archetypical result here is that the superlevel sets of the first eigenfunction of the Dirichlet Laplacian are convex. We’ll look at a few different proof techniques (maximum principles, constant-rank theorems) as well as some open problems in the field.
YouTube – Playlist: https://youtube.com/playlist?list=PLtqispdPhccZ2OLRJZcv3v_qmlVCCTFqd
———-
Title: Introduction to Mathematical Control Problems
Luz de Teresa, Federal University of Paraíba and Universidad Nacional Autónoma de México
Abstract: In this minicourse, I will introduce some control problems that are treated from the mathematical point of view. We will present some results and techniques related with Ordinary and Partial Differential Equations.
YouTube – Playlist: https://youtube.com/playlist?list=PLtqispdPhccYylVWwaZfubHqBjAV9EKRh
———-
Title: Regularity results for elliptic equations involving regional fractional Laplacian
Mouhamed Fall, African Institute for Mathematical Sciences
Abstract: We consider nonlocal operators generated by symmetric stable processes describing motions of random particles in a region Omega which are censored to jump outside Omega. While particles can jump inside the domain, they are either reflected inside or killed when they reach the boundary of the domain. Atypical examples of such operators are the “regional fractional laplacians”. In the Brownian case these phenomenon can be described by the Laplace operator subject to Neumann or Dirichlet boundary conditions. A natural generalization to symmetric stable Lévy processes was found by Bogdan, Burdzy and Chen. In this lecture, we shall investigate the properties of these nonlocal operators and the regularity results of solutions to the corresponding Poisson problem.
———-
Title: Spontaneously stochastic solutions
Alexei A. Mailybaev, IMPA
Abstract: Models of Fluid Dynamics that lack uniqueness of solutions arise in studies of turbulence. We consider such models within a broader context of differential equations that lack Lipschitz continuity and, therefore, require a regularization procedure or a selection criterion for defining relevant solutions. Starting with simplified models, we show how spontaneously stochastic solutions arise in formally deterministic systems. This phenomenon occurs when one takes into account an infinitesimal noise in the regularization process. Then we present theoretical and numerical results demonstrating spontaneous stochasticity in realistic models of fluid dynamics.
———-
Title: Nonlocal Hénon equation in R^N
Alexander Quaas, Universidad Técnica Federico Santa María
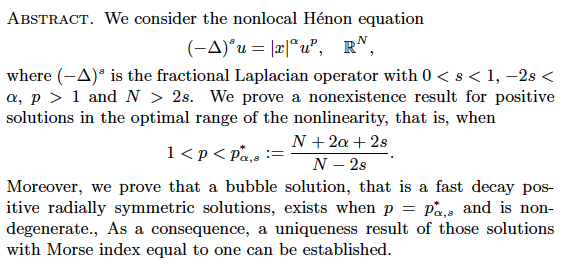
YouTube: https://www.youtube.com/watch?v=4FoXovqpkno
———-
Title: A broad look at elliptic partial differential equations
Enrico Valdinoci, University of Western Australia
Abstract: We will discuss some classical (and less classical) topics related to elliptic partial differential equations, related also to symmetry, classification and regularity theory. Some applications arising in geometry and material sciences will be also presented.
YouTube – Playlist: https://youtube.com/playlist?list=PLtqispdPhccaGD0aTCRmRdsaFpdpR_GmH






