Title: Stationary fully nonlinear mean-field games
Pêdra Andrade, CIMAT
Abstract: In this talk, we examine fully nonlinear mean-field game systems. First, we combine the regularity theory for fully nonlinear problems with the information on the double-divergence equation to produce gains of integrability/regularity for the solutions of the MFG system. Second, we prove the existence of minimizers for the variational problem and the existence of solutions to the mean-field games system. We also investigate a unidimensional example and unveil new information on the explicit solutions. This is joint work with Edgard Pimentel.
Youtube: https://www.youtube.com/watch?v=XkfYx9A3-QA
———-
Title: Sobolev Type Inequality for Intrinsic Varifolds
Julio Cesar Correa, PUC-Rio
Abstract: The lack of compactness in geometric variational problems involving the area functional leads to investigate a boarder class of geometric objects. In the early 70’s the (integral) varifolds was introduced as a class of “geometric” objects with compactness properties. Those varifolds are suitable generalization of the classical surfaces which permits “singular points” (in the sense of differential geometry).
The compactness of the class of (integral) varifolds allows to apply the direct method of the calculus of variations to the problem of minimize the area functional. Thus the regularity of those (integral) varifolds minimizing area is a natural question. Regularity of (integral) varifold is usually studied by an approximation by Lipschitz (single or multivalued) functions, a basic property of those functions is the Sobolev Inequality.
In this work we examine varifolds intrinsically defined in a given Riemannian manifold with bounded geometry, and we prove the monotonic behavior of its density ratio. This result leads to a version of a Sobolev type inequality, in which, surprisingly, the Sobolev constant does not depend on the geometry of the varifold.
YouTube: https://www.youtube.com/watch?v=C-H2axnHTkk
———-
Title: Existence Of Steady States For The Maxwell-Schrödinger-Poisson System: Exploring The Applicability Of The Concentration-Compactness Principle
Gabriel N. Cunha, IME-UFG
Abstract: In this talk, I’m going to discuss the main ideas concerning a variational approach for the M.S.P system using the concentration-compactness principle presented by P.l.lions in his famous paper on this subject. This discussion is based on the paper by I.Catto, J-Dolbeaut,O.Sáches and J-Soler, with the same title.
YouTube: https://www.youtube.com/watch?v=Qs_RmberWAs
———-
Title: Radial solutions for a class of Hénon type systems with partial interference with the spectrum
Eudes Mendes, UFRPE
Abstract: We investigate the existence of radial solutions for a class of Hénon type systems with nonlinearities reaching the critical growth and interacting with the spectrum of the operator with the possibility of double resonance. The proof is made using variational methods, combining Brézis and Nirenberg arguments with Ni compactness result and Rabinowitz linking theorem.
YouTube: https://www.youtube.com/watch?v=qUlGFnieCcY
———-
Title: Finer analysis of the Nehari set associated to a class of Kirchhoff-type equations
Steffanio Moreno, IME-UFG
Abstract:

YouTube: https://www.youtube.com/watch?v=x4AQtu3xg-Q
———-
Title: A dynamical system approach to a class of radial Henon-Lane-Emden type equations of fully nonlinear type
Gabrielle Nornberg, ICMC-USP
Abstract: In this talk we discuss existence, nonexistence and classification of radial positive solutions of a class of weighted nonlinear equations of Lane-Emden type. Our approach is entirely based on the analysis of the dynamics induced by an autonomous quadratic system, which is obtained after a suitable transformation.This method allows to treat both regular and singular solutions in a unified way, without using energy arguments. We also compare known results of the standard Henon-Lane-Emden equation and differences produced when one replaces the Laplacian operator by a Pucci fully nonlinear operator. Based on a joint work with Liliane Maia (Universidade de Brasília) and Filomena Pacella (La Sapienza Università di Roma).
YouTube: https://www.youtube.com/watch?v=-PHlIsUfI_s
———-
Title: A fully nonlinear free transmission problem
Makson Santos, CIMAT
Abstract: We study the regularity of the solutions and some geometric properties of the free boundary of a free transmission problem driven by fully nonlinear elliptic operators. By relating our problem with a pair of viscosity inequalities, we prove that the gradient of strong solutions are Lipschitz, locally. As regards the free boundary, we start by establishing weak results, such as its non-degeneracy, and proceed with the characterization of global solutions.
YouTube: https://www.youtube.com/watch?v=HsvAV5Tq6vw
———-
Title: Ground states for cooperative Schrödinger type equations coupled with local and nonlocal nonlinearities
Gaetano Siciliano, IME-USP
Abstract: We consider an Hartree-Fock type system made by two Schrödinger equations in presence of a Coulomb interacting term and a cooperative pure power and subcritical nonlinearity, driven by a suitable positive parameter beta. We show the existence of semitrivial and vectorial ground states solutions depending on the parameters involved. The asymptotic behavior with respect to the parameter beta of these solutions is also studied.
YouTube: https://www.youtube.com/watch?v=gVP6OWY_IFU
———-
Title: Improved regularity results for a class of nonlocal L_0 – Elliptic equations through asymptotic profiles
Aelson Sobral, UFPB
Abstract: In this talk we present new regularity results for a class of nonlocal L_0 – elliptic equations with an asymptotic property. We start by defining the nonlocal version of the recession operator, discuss the main features and compare it with its classical version. The role of this class of equations is detailed on some examples and an improved regularity result.
YouTube: https://www.youtube.com/watch?v=Ljz_qiC5QNU
———-
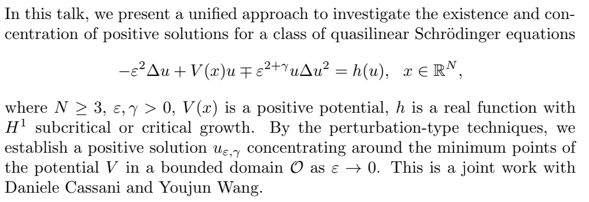
Title: A unified approach to a class of quasilinear Schrodinger equations
Jianjun Zhang, Chongqing Jiaotong University
Abstract: